calgarycros.blogg.se

1. #MOMENT OF INERTIA CALCULATOR T BEAM HOW TO#
2. #MOMENT OF INERTIA CALCULATOR T BEAM PLUS#
3. #MOMENT OF INERTIA CALCULATOR T BEAM FREE#

The crotch thickness is measured at the outside intersection of the web and the flange. Tapered beams have tapered web and tapered flanges. This tool calculates the section modulus, one of the most critical geometric properties in the design of beams subjected to bending.

Adapting the basic formula for the polar moment of inertia (10.1.5) to our labels, and noting that limits of integration are from 0 to r, we get. Semi tapered beams have a straight web with tapered flanges. The differential area of a circular ring is the circumference of a circle of radius times the thickness d. The Tee section is assumed to be symmetrical along axis 2 (the flanges are equal length and thickness). mm A Paint area per unit length of profile. Help Using The Pipeng Toolbox (opens in the popup workbook) Links : ±ĬALCULATOR : Beam Cross Section Properties (T Section Beam) ±Ĭalculate T section beam cross section properties for straight sided beams, semi tapered T beams, and tapered T beams. Properties of standard beams t Thickness or width of the vertical element(s).

#MOMENT OF INERTIA CALCULATOR T BEAM PLUS#

Try plus mode using the Plus Mode Demo tools with no login. Buy a Subscription to use the tools in plus mode (with plots, tables, goal seek etc).

#MOMENT OF INERTIA CALCULATOR T BEAM FREE#

tools are free in basic CHECK mode with Login or Open a free account (CHECK values no plots, tables, goal seek etc). Login or Open a free account to use the tools in plus mode (with plots, tables, goal seek etc). tools are free in basic mode with no login (no plots, tables, goal seek etc). Triangular Beam Cross Section Calculation Module.Trapezoid Beam Cross Section Calculation Module.Square Beam Cross Section Calculation Module.Right Angle Beam Cross Section Calculation Module.Rectangular Channel Beam Cross Section Calculation Module.Rectangular Beam Cross Section Calculation Module.Polygon Beam Cross Section Calculation Module.Parallelogram Beam Cross Section Calculation Module.I Beam Cross Section Calculation Module.

#MOMENT OF INERTIA CALCULATOR T BEAM HOW TO#

Elliptical And Semi Elliptical Beam Cross Section Calculation Module Moment of inertia finding moment of inertia or area of moment of inertia of T section.This tutorial will make you fully understand how to calculate the area.Diamond Beam Cross Section Calculation Module.Circular Pipe Cross Section Calculators.Circular And Semi Circular Beam Cross Section Calculation Module.Beam Section Modulus Calculation Module Section modulus is used in structural engineering to calculate the bending moment that will result in the yielding of a beam with the following equation: where S section modulus y material’s yield strength Beams in bending experience stresses in both tension and compression.Beam Cross Section Parallel Axis Theorem Calculation Module.Beam Cross Section Concrete Stiffness Factor Calculation Module.Beam Cross Section Added Mass Calculation Module.

Reference : Roark's Formulas For Stress And Strain, Warren C Young, McGraw Hill Change Module : This calculator is developed to help in determination of moment of inertia and other geometrical properties of plane sections of beam and column.

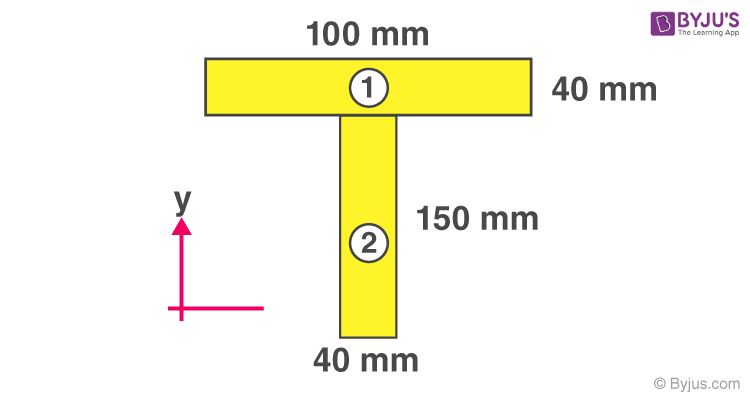
The Tee section is assumed to be symmetrical along axis 2, and the flanges are assumed to be equal length and thickness. The distance of each piece of mass dm from the axis is given by the variable x, as shown in the figure.Calculate beam cross section properties for T section beams: cross section area, moment of inertia, polar moment of inertia, mass moment of inertia, section modulus, radius of gyration, EI, EA, EAα, unit mass, total mass, unit weight and specific gravity. We can therefore write dm = \(\lambda\)(dx), giving us an integration variable that we know how to deal with. Note that a piece of the rod dl lies completely along the x-axis and has a length dx in fact, dl = dx in this situation. We chose to orient the rod along the x-axis for convenience-this is where that choice becomes very helpful. If we take the differential of each side of this equation, we find